Polarizer
Polarizer ya da polarizing filter, üzerine gelen ışının elektrik alanının belirli bileşenini filtreliyor, öteki tarafa geçirmiyor; diğer bileşenini geçiriyor. Filtrelemeyi, polarizer kendi bünyesine alarak yapıyor, absorb ediyor. Gelen ışının belirli doğrultudaki elektrik alanı, polarizer'ın elektronlarını harekete geçirebiliyor (kinetik enerjiye dönüştü demektir). Böylelikle ışının bir miktar enerjisi, polarizer tarafından absorblanmış oluyor.
(Electric field energy \(\Longrightarrow\) Kinetic energy \(\Longrightarrow\) Heat)
Elimizde unpolarized light olsun. Işığın zaman içinde değişen elektrik alan vektörlerinin (\(\vec{E}\)) her birini birbirine dik 2 eksene izdüşürebiliriz. \(\vec{E}\) 'ler randomly oriented. Randomly oriented'ın istatistiksel olarak tüm doğrultularda eşit miktarda bulunduğu şeklindeki yorumunu kabul edelim.

\(I_0 \propto E^2\)
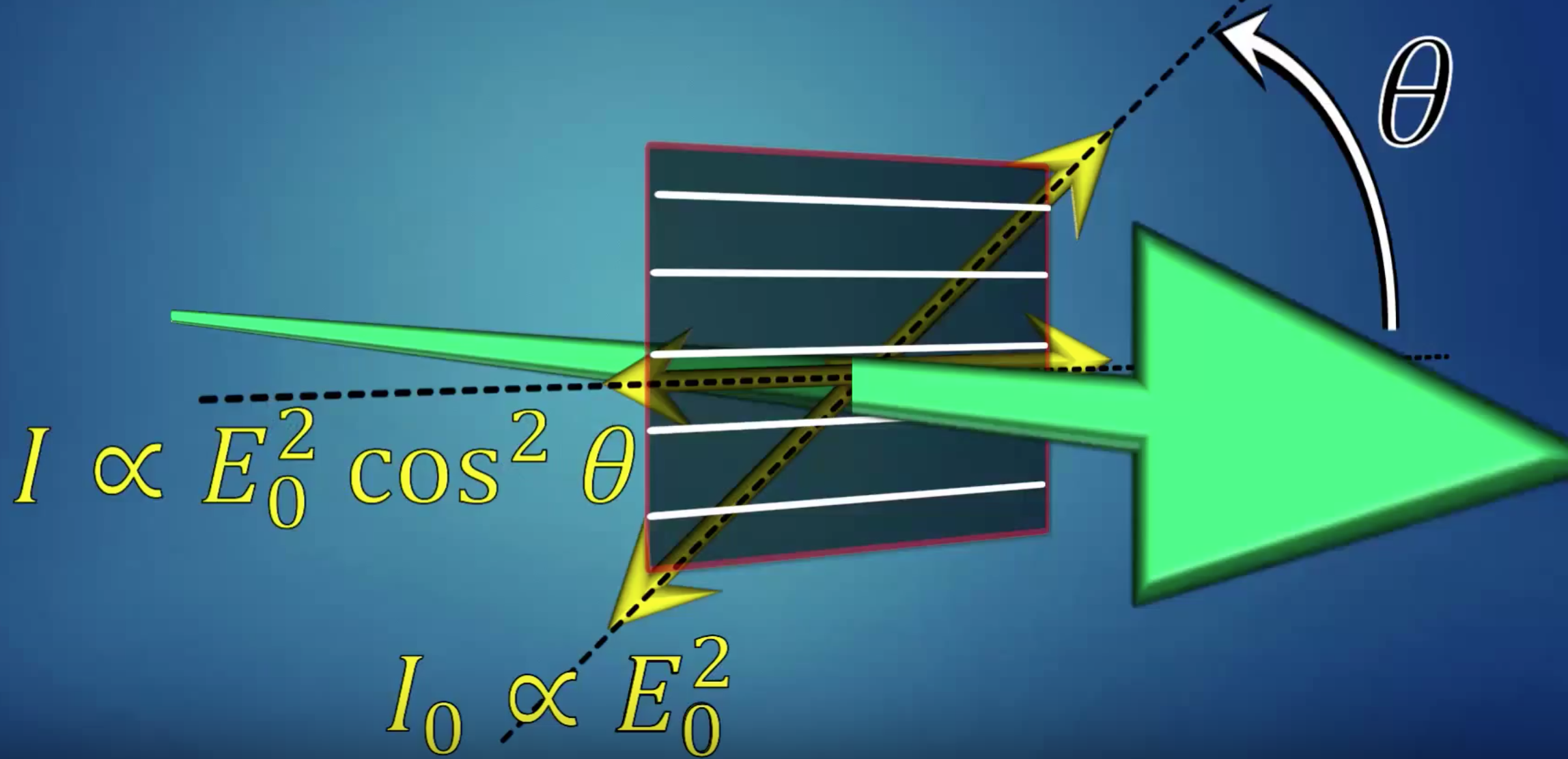
Gelen ışın eksenlerin biriyle belirli bir açı yapsın: \(\theta\). Böylelikle her eksene düşen \(\vec{E}\) hesaplanabilir. Eksenlerin birine \(\vec{E}cos\theta\), diğerine \(\vec{E}sin\theta\) kadar düşer; ışın şiddetleri de \((\vec{E}cos\theta)^2\) ve \((\vec{E}sin\theta)^2\) olur.
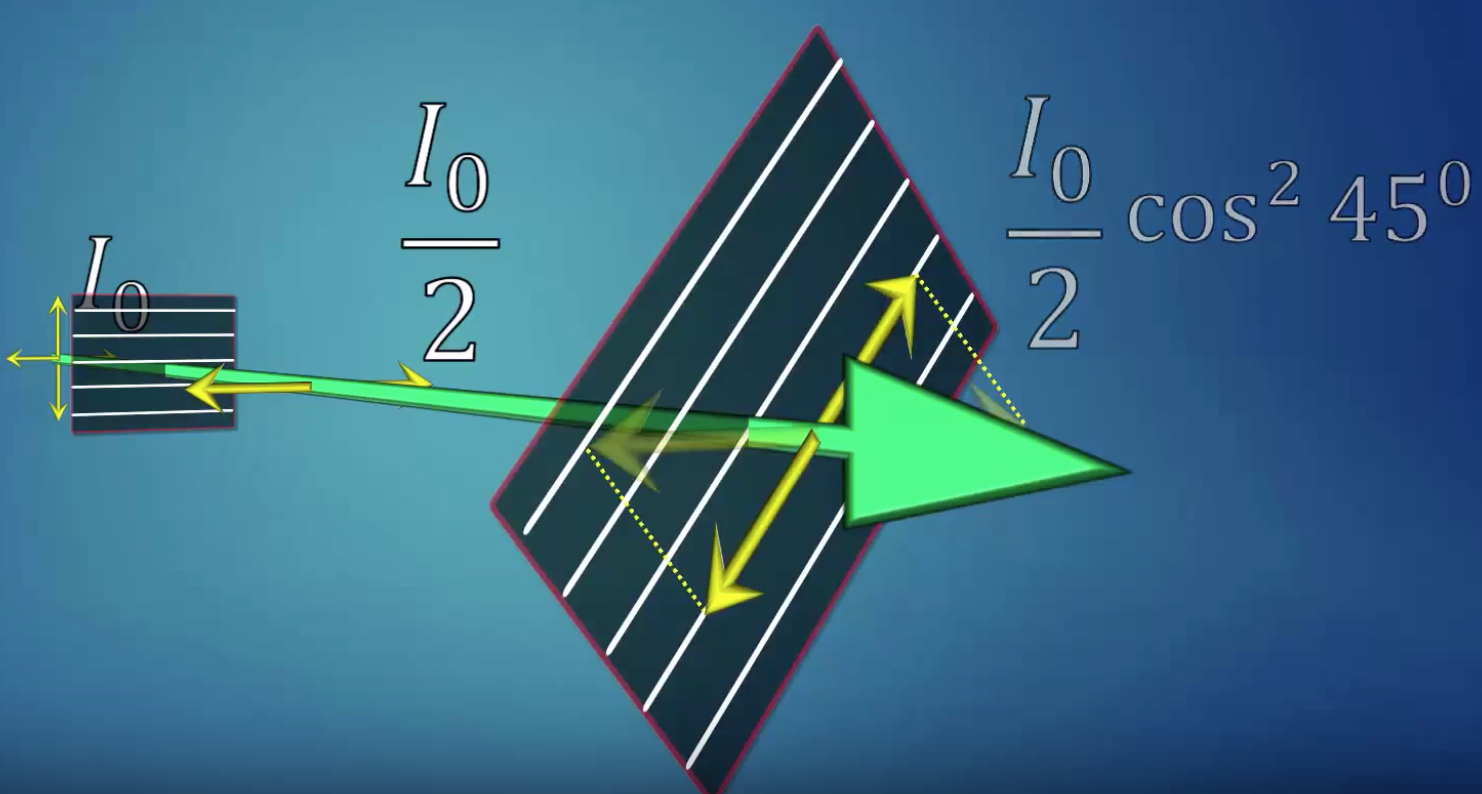
Gelen ışın, eksenlerle \(45^\circ\) 'lik açı yaparsa eksenlerin her birine düşen: \(E^2sin^2\theta=E^2(\frac{1}{\sqrt{2}})^2=\frac{E^2}{2} \propto \frac{I_0}{2}\)
Polarizing Filter
Polymer: Kendisinden küçük, tekrar eden yapılardan oluşan molekül. Polymers are composed of smaller repeating units called monomers.

Crystal: Katı materyal. 3 boyutta tekrar eden yapılardır. Materyalin içeriği moleküller, atomlar ya da iyonlar.
Polarizing filter: Nitrocellulose polymer film'e gömülmüş (embed) microscopic iodiquinine sulphate (herapathite) kristalleri. Üretim sırasında kristaller, iğne şekline (needle-like crystals) getirilirmiş. Metot: stretching ya da elektrik veya manyetik alan uygulamakmış.

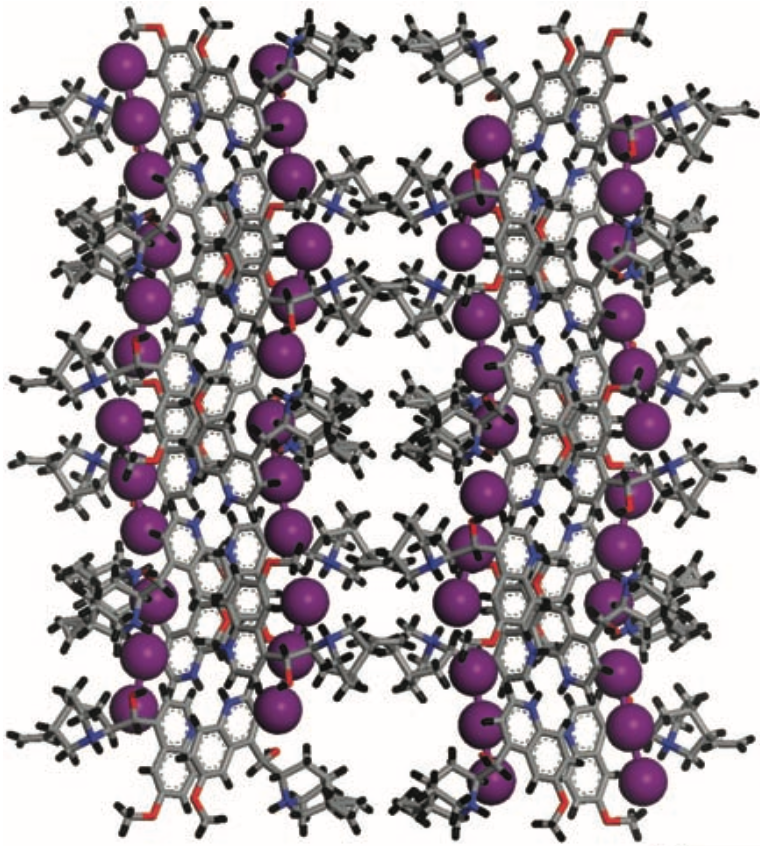
Üstteki resim Herapathite'tın yapısı. Mor küreler iodine atomları. The absorbing axis is vertical.
Bu eksenlerden birine paralel olacak şekilde absorbing axis'i koyarsak ışının o eksene paralel bileşeni absorbe edilir ve materyalden çıkamaz. Demek ki polarizer'a gelen ışının absorbing axis'e paralel bileşeni emilir, ışının absorbing axis'e dik bileşeni kurtulur. Böylelikle gelen ışının polarizasyonu çıkarken değişir.
Bu örnekte polarizer, ışığın şiddetinin azalmasıyla ilgili. Absorblama elektronların hareket edebilmesiyle ilgili, elektronlara kinetik enerji aktarılabilmesiyle ilgili.
Polarizasyonun bir ekseni absorbing axis, diğer ekseni transmission axis.
Tek doğrultuda polarizasyona sahip (linearly polarized) bir ışını transmission axis'la \(90^\circ\) 'lik açı yapacak şekilde ayarlamadığın sürece ışının polarizasyonunu döndürebilirsin. Ta ki ışın şiddeti gözle görülene kadar.
Aşağıdaki resimlerde polarizer'ın transmission axis'ını görüyoruz.
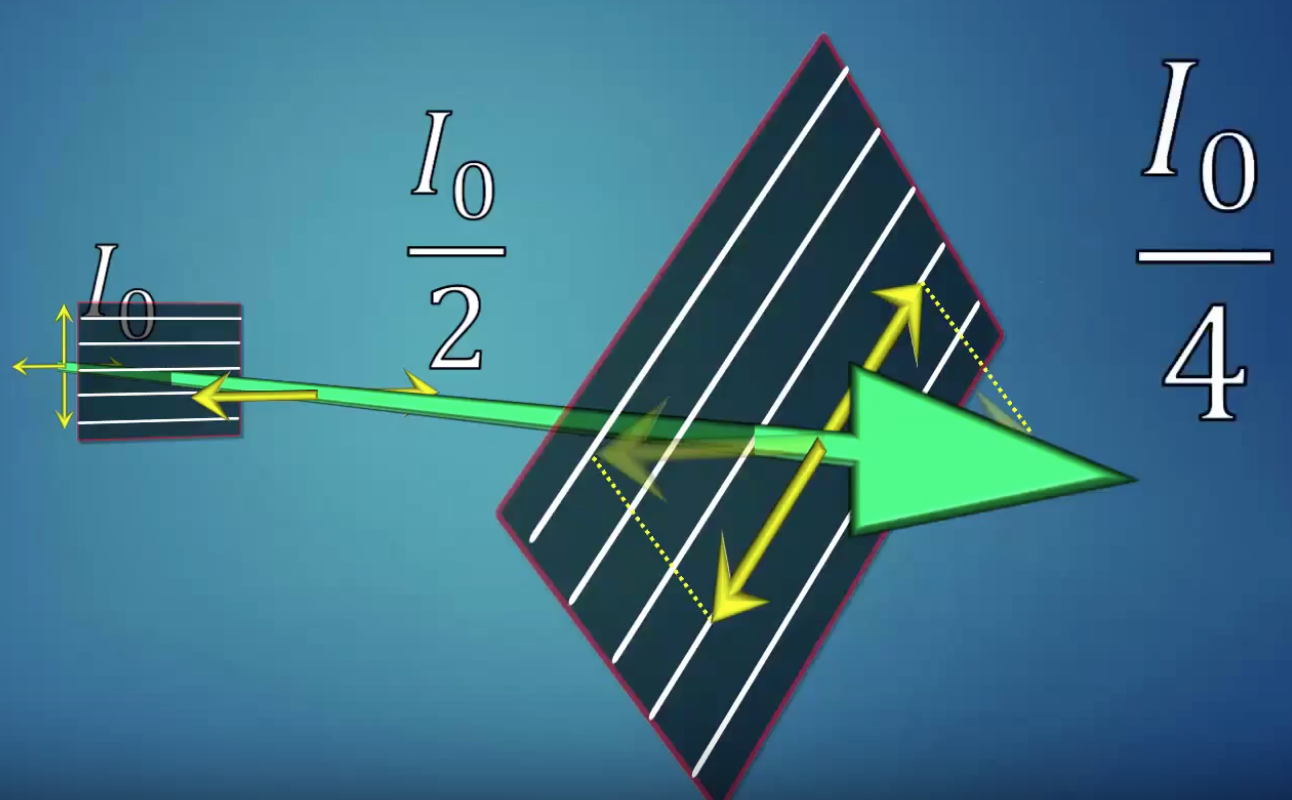
Polarizing Beam Splitter
PBSs split the incident beam into 2 beams. Two types of PBS: 2 beams perpendicular to each other or 2 beams with an angle between \(15-45^\circ\).
Physics behind the \(90^\circ\) type of PBS:
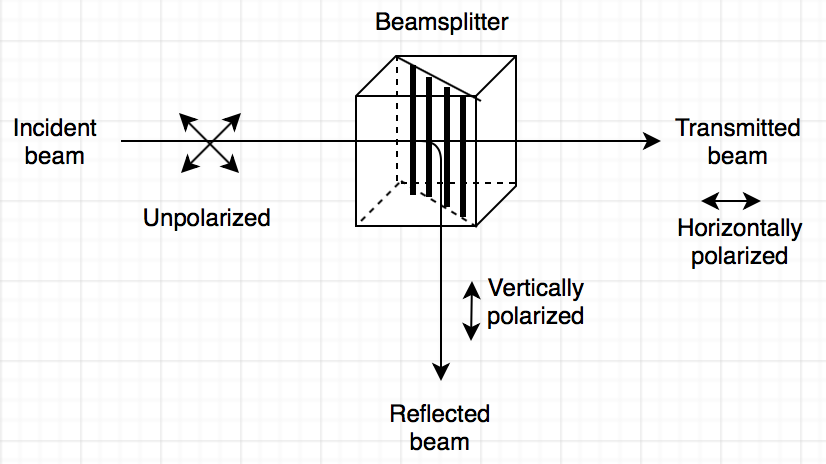
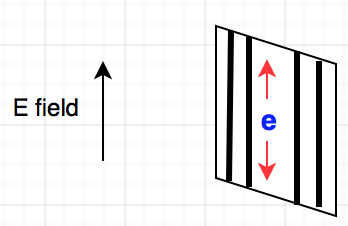
- Electrons are oscillating in the metal wires. The electrons of the interface have the ability to move in a specific direction. Let's say incident beam is unpolarized, namely, the electric field of it has components of both parallel and perpendicular to the direction of electron movement. The parallel component enables electrons to move. The perpendicular component doesn't affect the electrons' movement. So parallel component is absorbed by the electrons. Since the perpendicular component does't have the ability to drive electrons, this component transmits the interface and the PBS. After the absorbance, electrons radiate energy. I assume that this energy has the same polarization, frequency and phase with the incident beam. How about the determination of the propagation direction of the reflected beam?
Hertzian dipole model:
Two spherical conductors are wired. Electric charge flow is periodically back and forth. When one conductor has \(q(t)\) charge, the other has \(-q(t)\).
\(q(t)=q_0 \sin{\omega t}\)
Oscillating current:
\(I(t)=\frac{dq}{dt}=I_0 \cos{\omega t}\), \(I_0=\omega q_0\).
Magnetic vector potential generated by a current distibution \(j(r)\):
\(A(r,t)=\frac{\mu_0}{4\pi}\int\frac{j}{|r-r'|}d^3r'\).
We assume that wire is aligned along the z-axis from \(z=-\frac{l}{2}\) to \(z=\frac{l}{2}\), wire's thickness is negligible, we calculate for the region \(r>>\lambda >>l\) where \(\lambda=\frac{2\pi c}{\omega}\) is the wavelength of the emitted radiation generated by the oscillating current. And ultimately, it takes the form:
\(A_z(r,t)=\frac{\mu_0 l}{4\pi}\frac{I(t-r/c)}{r}\).
The scalar potential is evaluated using the Lorentz gauge condition:
\(\nabla\cdot A=-\epsilon_0\mu_0\frac{\partial \phi}{\partial t}\).
After we calculate the vector and scalar potentials, we evaluate \(E\) and \(B\) fields:
\(E=-\frac{\partial A}{\partial t}-\nabla\phi\),
\(B=\nabla\times A\).
Radiation fields are calculated as
\(E=-\frac{\omega l I_0}{4\pi\epsilon_0 c^2}\sin{\theta}\frac{sin{[\omega(t-r/c)]}}{r}\hat{\theta}\)
\(B=-\frac{\omega l I_0}{4\pi\epsilon_0 c^3}\sin{\theta}\frac{sin{[\omega(t-r/c)]}}{r}\hat{\varphi}\)
\((r, \theta, \varphi)\) are spherical polar coordinates. \(\varphi\) is azimutal angle.
I think, in the determination of the propagation direction, we utilise the arranging of the atoms/molecules in the interface. Photon or light can bounce from a surface. It has different property from an ordinary electromagnetic (EM) wave. EM wave can not bounce from asurface but light can bounce. Maybe, we utilise the property of the light and the arrangement of the molecules of interface. Polarization of the reflected beam is perpendicular to the propagation direction so the it is in a plane perpendicular to the propagation direction.
- External E field causes electrons to move. On the other hand, electrons create their own E field. And there is voltage (voltage difference). Voltage difference cause electron movement in order to reduce the electric potential energy. In the existance of voltage difference, one side has positive voltage, the other side has negative voltage. Positive voltage is attraction force. Negative voltage is pusher force. As electrons move in a diection in order to reduce the electric potential energy.